Research at the Laboratory of Crystallography
The structure of the 2-dimensional Charge-density wave in (PO2)4(WO3)8
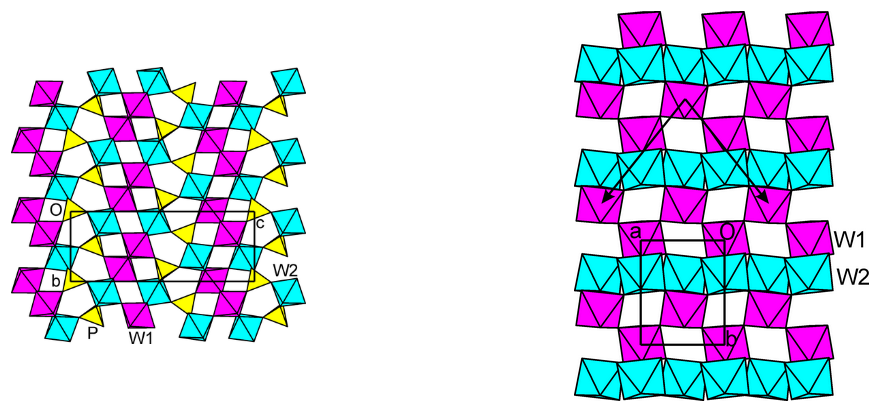
Fig. 1: Projection of the basic structure of (PO2)4(WO3)8 (left) along a clearly shows the WO3 layers and the PO4 tetrahedra with pentagonal tunnels, and (right) along c shows zigzag chains of vertex-sharing W1 octahedra parallel a, while chains of vertex-sharing tungsten octahedra with the repeat unit (W1-W1-W2) can be recognized parallel a + b as well as parallel -a + b. The two crystallographically independent tungsten atoms W1 and W2 are indicated by purple and green octahedra, respectively. Yellow tetrahedra represent the PO4 groups.
The monophosphate tungsten bronzes with pentagonal tunnels (PO2)4(WO3)2m comprise a homologous series of compounds with m = 4 or larger. The crystal structures are built of conducting slabs of WO3 with a ReO3-type structure separated by layers containing the phosphate groups (Fig. 1). The layers support 2-dimensional (2D) electron bands, and their thickness is proportional to m. Studies of the temperature dependences of their electical and magnetic properties as well as of diffuse scattering phenomena have shown that at low temperatures charge-density waves (CDWs) develop in these compounds. The CDW properties depend on m, too, whereby higher transition temperatures are found for compounds with larger m.
The m = 4 compound (PO2)4(WO3)8 has CDW transitions at Tc1 = 80 K and Tc2 = 52 K. We have studied these CDWs by x-ray scattering at various temperatures, using synchrotron radiation at beamline D3 of Hasylab at DESY, Hamburg. In a first experiment, the positions of the main reflections and the satellite reflections were measured at temperatures between 20 K and 90 K [1]. It was found that the lattice remained orthorhombic down to T = 20 K with lattice parameters a = 5.294 (1) A, b = 6.591 (2) A, and c = 17.415 (16) A. The primary CDW that develops below Tc1 could be described as a 2D CDW with modulation wave vectors:
q1 = (0.330(2), 0.292(2), 0.000(2))
q2 = (-0.330, 0.292, 0.000)
q1 and q2 are related to each other by the orthorhombic symmetry.
Below the second transition Tc2 additional satellite reflections develop, that were found to correspond to mixed second-order satellites of the primary CDW, given by the wave vector
q1-q2 = (0.660, 0, 0).
A consequence of these observations is that all Bragg reflections can be indexed with 5 integers (h, k, l, m1, m2 according to
H = h a* + kb* + l c* + m1 q1 + m2q2
The main reflections have indices (h, k, l, 0, 0). The satellite reflections corresponding to the primary CDW are defined by (m1, m2) = (±1, 0) or (0, ±1). The secondary CDW corresponds to the satellites with (m1, m2) = ±(1, -1). Integrated intensities of these reflections were measured at T = 20 K. From these data, the modulated structure was determined by the superspace method [2]. The modulation functions are described by Fourier series, with the harmonic coefficients (1, 0) and (0, 1) defining the primary CDW and the harmonic coefficients (1, -1) defining the secondary CDW. The analysis of the modulated structure lead to establishment of the following characteristics of the CDW:
- The CDW originates in low-dimensional electron bands on the tungsten atoms of the WO3 layers.
- The CDW is 2-dimensional, and it remains this way throughout the whole temperature range below Tc1 = 80 K.
- The CDW corresponds to the formation of clusters of 6 tungsten atoms, that are linked together to form superclusters of 12 to 18 atoms (Fig. 2).
- The second CDW transition at Tc2 = 52 K corresponds to the development of the mixed second order modulations rather than an independent CDW. Its effect is to further stabilize the clusters of W atoms.
- The presence of clusters of tungsten atoms stresses the 2D character of the CDW, as it is opposed to a collection of one dimensional CDWs residing on different chains of vertex sharing WO6 octahedra. The relation with the CDWs in transition metal dichalcogenides is noted, for which clusters of metal atoms have been established as the characteristic feature of the CDW [3, 4].
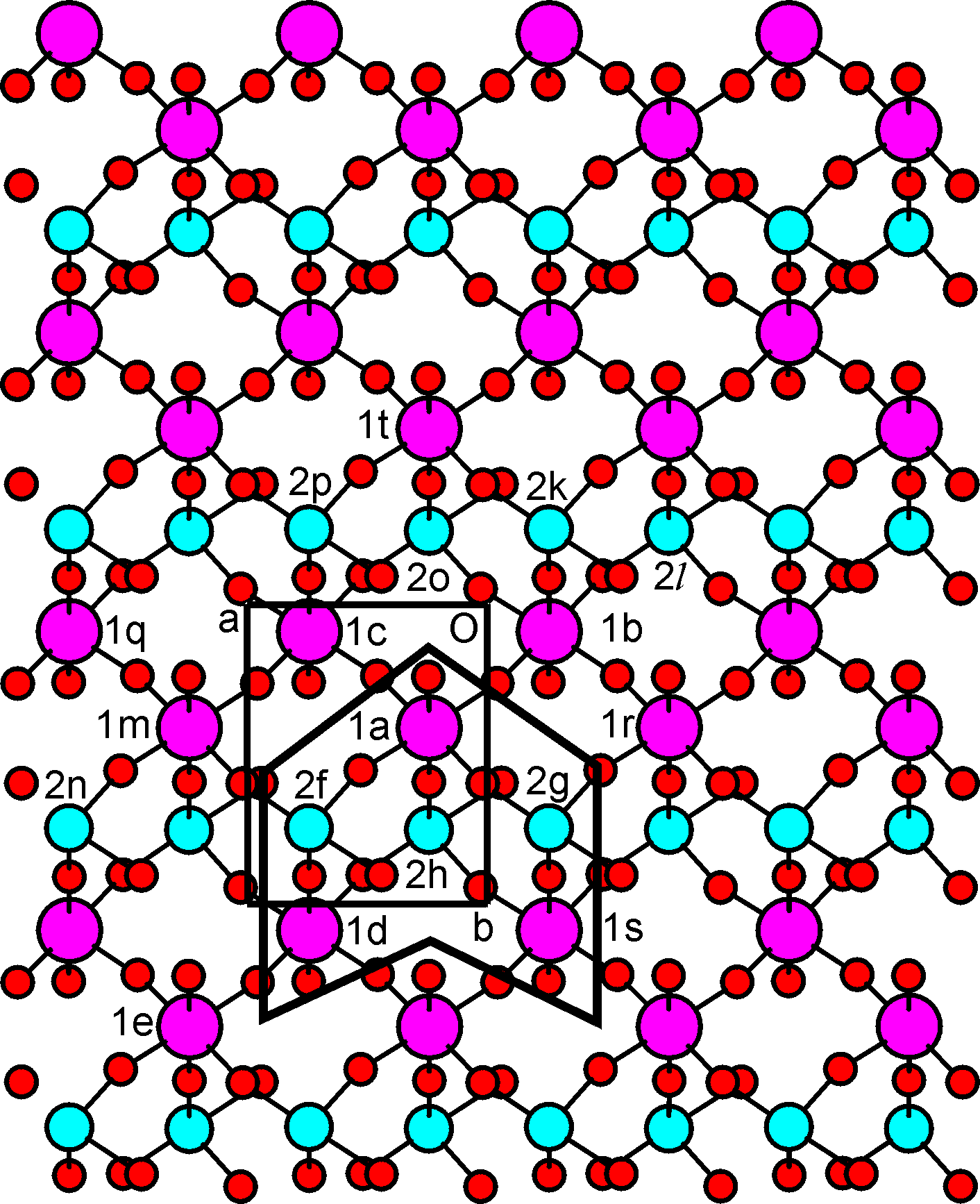
Fig. 2: Projection of one layer of WO3 onto the (a, b) plane, showing the individual atoms. Large and medium sized circles represent W1 and W2 atoms, respectively. Small circles represent oxygen. Phosphor is not shown. The unit cell and the fundamental cluster unit of 6 tungsten atoms are indicated by thick lines.
Literature:
[1] J. Lüdecke, A. Jobst, and S. van Smaalen (2000): The CDW structure of the m = 4 phosphate bronze (PO2)4(WO3)2m. Europhys. Lett 49, 357.
[2] J. Lüdecke, A. Jobst, S. Geupel, and S. van Smaalen (2001): The structure of the two-dimensional, incommensurate charge-density wave in(PO2)4(WO3)8. at T = 20 K. Phys. Rev. B 64, 104105.
[3] A. Spijkerman, J.L. de Boer, A. Meetsma, G.A. Wiegers and S. van Smaalen (1997): X-ray crystal structure refinement of the nearly-commensurate phase of 1T-TaS2 in (3+2)-dimensional superspace. Phys. Rev. B 56, 13757.
[4] J. Lüdecke, S. van Smaalen, A. Spijkerman, J. L. de Boer, and G.A. Wiegers (1999): The commensurately modulated structure of 4Hb-TaSe2 determined by X-ray crystal structure refinement. Phys. Rev. B 59, 6063.
